I saw this problem earlier today, and really enjoyed it. I realize it's an oldie, but had never seen it before. And I wanted to see what you guys thought of it.
Oh, and I've tweeked it to make it Trek appropriate.
So, what say you?
Oh, and I've tweeked it to make it Trek appropriate.

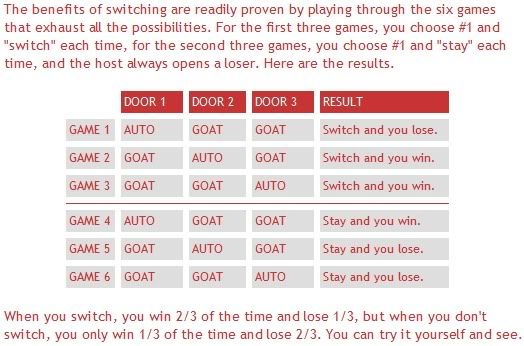
Let's say that you're on a game show, and you're given the choice of three doors. Behind one door is an Orion Slave Girl, behind the others, Klingon Warriors. You pick a door, say #1, and the host, who knows what's behind each of the doors, opens another door, say #3, which has a Klingon. He says to you, "Do you want to change to door #2?" Do you think that it is to your advantage to switch your choice of doors?
So, what say you?