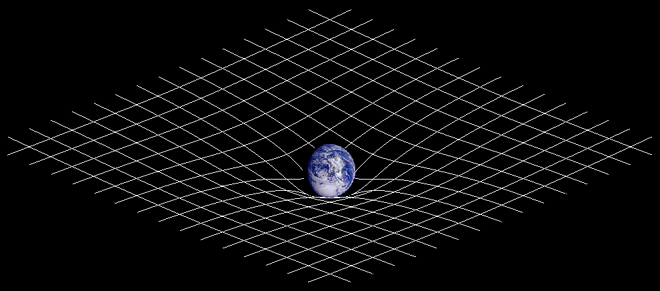
Our Universe, as you may know, is a fabric. A fabric made from the fibers of time and the fibers of space.
Space and time are the two fundamental ingredients of the Universe, and have many things in common. We move inside them. We cannot understand a position in them without relating it with another position. Both can be measured.
But, while in space we can move in 3 dimensions, in time we can only move forward, and so time is considered itself a dimension.
Now, as we know from the String Theory, there are actually more dimensions than the ones we can move in. In fact, there are 11 dimensions, including time. The reason we cannot move in those extra dimensions, is that they are extremely small. Only some nanometers "wide", and everything has the same "width" in them.
Imagine two little people drawn on a piece of paper. They are three-dimensional, but they can only move in two dimensions. That is because the third dimension for them is the fatness of the paper. But it is very small a dimension, and equal for both of them.
Though, extremely small particles like Qwants seem to be able to move in more dimensions than just 3 in space. Also, the reason Gravity is by far the weakest force in the Universe, is also because it is scattered in all dimensions, according to some theories.
How weak is gravity, you ask? Take a paperclip, and let it fall on the floor. The whole planetpulls it toward its center, with the force of gravity. Now tak a small magnet, and take it close to the paperclip. The magnet immediately pulls it up, and now the paperclip is stuck on the magnet. So, a small magnet's magnetic force is able to surpass by far the gravity force of a whole planet. Gravity is THAT weak.
The String Theory was recently proven wrong, but the fact that there are more dimensions in space we cannot see, remains. Also, according to the Brane Theory, a branch of the String Theory, another Universe could be as close to us as some centimeters, but in a direction in one of the dimensions that are hidden from us.
Another scientific breakthrough was the Multiverse theory. This theory states that there is an infinite number of Universes outside ours. Those Universes can be as different than ours as Tiny Toons from reality, and as close to ours as you deciding not to read this post being the only difference.
In fact, a branch of the Multiverse theory states that each Universe is a branch of our timeline, where you took another decision. For instance, when you are in a crossroad, you decide to go left, but in another Universe, you decide to go right. There is a Universe for every possibility. Everything that could happen, has happened, and will happen, somewhere in the Multiverse.
But, as we perceive it, time is linear. That means we can follow only one path, with another "us" following a different path in another Universe. The truth is, time is not linear at all, but we can see only one of its paths.
Does that remind you of something?
Is it not the same in space with its dimensions?
We consider time a dimension, because we can only move in one dimension in it.
But here I would like you to read some lines of the book "Flatland", by Edwin Abbot Abbot. In these lines, a two-dimensional creature is trying to understand an one-dimensional creature.
"-I am a line, the longest in Lineland, over three inches of space-" "Of Length," I ventured to suggest. "Fool," said he, "Space is Length."
Could it be possible, then, that time has its own dimensions, and we cannot understand them because we live in only one of them? In time, we are a zero-dimensional dot, moving in an one-dimensional line. But could it be more than that in time? Could those other timelines, with infinite decisions, be considered a proof that time is multi-dimensional, instead of one-dimensional? And if so, what implications could this theory have in science? Maybe, if that is the case, we could be able to find a "Geometry of time", or Chronometry if you will, that could possibly help us predict what could be in other Timelines, and how far from our Universe are our other decisions...
If not, why?
What do you think?
Space and time are the two fundamental ingredients of the Universe, and have many things in common. We move inside them. We cannot understand a position in them without relating it with another position. Both can be measured.
But, while in space we can move in 3 dimensions, in time we can only move forward, and so time is considered itself a dimension.
Now, as we know from the String Theory, there are actually more dimensions than the ones we can move in. In fact, there are 11 dimensions, including time. The reason we cannot move in those extra dimensions, is that they are extremely small. Only some nanometers "wide", and everything has the same "width" in them.
Imagine two little people drawn on a piece of paper. They are three-dimensional, but they can only move in two dimensions. That is because the third dimension for them is the fatness of the paper. But it is very small a dimension, and equal for both of them.
Though, extremely small particles like Qwants seem to be able to move in more dimensions than just 3 in space. Also, the reason Gravity is by far the weakest force in the Universe, is also because it is scattered in all dimensions, according to some theories.
How weak is gravity, you ask? Take a paperclip, and let it fall on the floor. The whole planetpulls it toward its center, with the force of gravity. Now tak a small magnet, and take it close to the paperclip. The magnet immediately pulls it up, and now the paperclip is stuck on the magnet. So, a small magnet's magnetic force is able to surpass by far the gravity force of a whole planet. Gravity is THAT weak.
The String Theory was recently proven wrong, but the fact that there are more dimensions in space we cannot see, remains. Also, according to the Brane Theory, a branch of the String Theory, another Universe could be as close to us as some centimeters, but in a direction in one of the dimensions that are hidden from us.
Another scientific breakthrough was the Multiverse theory. This theory states that there is an infinite number of Universes outside ours. Those Universes can be as different than ours as Tiny Toons from reality, and as close to ours as you deciding not to read this post being the only difference.
In fact, a branch of the Multiverse theory states that each Universe is a branch of our timeline, where you took another decision. For instance, when you are in a crossroad, you decide to go left, but in another Universe, you decide to go right. There is a Universe for every possibility. Everything that could happen, has happened, and will happen, somewhere in the Multiverse.
But, as we perceive it, time is linear. That means we can follow only one path, with another "us" following a different path in another Universe. The truth is, time is not linear at all, but we can see only one of its paths.
Does that remind you of something?
Is it not the same in space with its dimensions?
We consider time a dimension, because we can only move in one dimension in it.
But here I would like you to read some lines of the book "Flatland", by Edwin Abbot Abbot. In these lines, a two-dimensional creature is trying to understand an one-dimensional creature.
"-I am a line, the longest in Lineland, over three inches of space-" "Of Length," I ventured to suggest. "Fool," said he, "Space is Length."
Could it be possible, then, that time has its own dimensions, and we cannot understand them because we live in only one of them? In time, we are a zero-dimensional dot, moving in an one-dimensional line. But could it be more than that in time? Could those other timelines, with infinite decisions, be considered a proof that time is multi-dimensional, instead of one-dimensional? And if so, what implications could this theory have in science? Maybe, if that is the case, we could be able to find a "Geometry of time", or Chronometry if you will, that could possibly help us predict what could be in other Timelines, and how far from our Universe are our other decisions...
If not, why?
What do you think?

 .
.

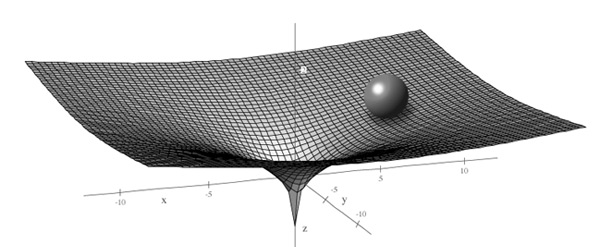
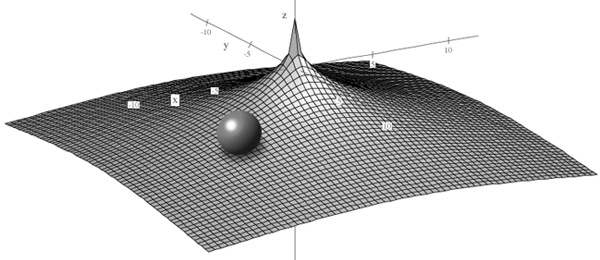

 . You are saying that from an external POV, the object in inertial motion in a gravitational field will change velocity aka accelerate/decelerate because it'll travel the same distance in a shorter/longer time interval than it would if the gravitational field was not there.
. You are saying that from an external POV, the object in inertial motion in a gravitational field will change velocity aka accelerate/decelerate because it'll travel the same distance in a shorter/longer time interval than it would if the gravitational field was not there.