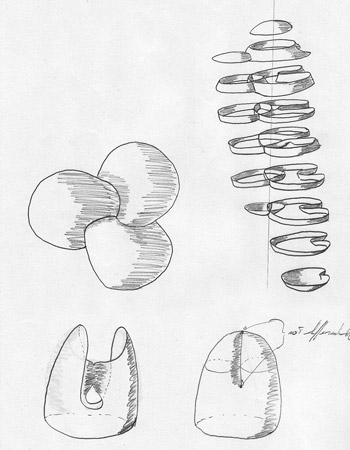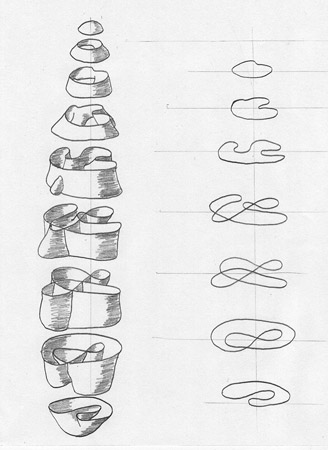I've been studying aspects of non-Euclidean geometries and topology since the late 1980s, and so it is sometimes hard for me to remember that most people aren't exposed to this stuff. And by most people, I'm including most people who major in math and physics too. These areas aren't pushed as mainstream topics even in math and physics, and are sometimes presented as being too hard for most people (even in those areas) to understand.
I, personally, think that with a little help these things can be understood... at least on a conceptual level. And a project was started back around 1990 to attempt to help people with visualizing this type of stuff. It started as an offshoot of the Minnesota Supercomputing Institute at the University of Minnesota called the Geometry Supercomputer Project. This was later given additional funding by the National Science foundation and the Department of Energy to form the Geometry Center.
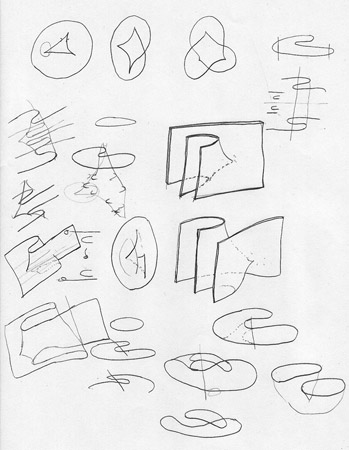
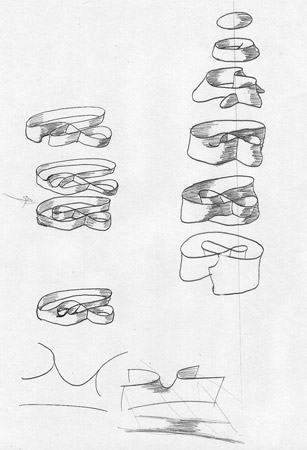
My connection with the Geometry Center was rather limited... I did research there in the summer of 1994 on the topic of tight immersions of manifolds while I was a student at UCSD. I had the odd distinction of being the only person there that summer doing research that didn't make use of the computer systems at the center (about 8 SGIs, 12 Suns, 30 NeXTs, and 25 Macs) for my research.
But the Geometry Center put out some great stuff that was design to be approachable by everyone. A couple of these projects included video productions, which I think might be interesting for some of the people here. And thankfully even after NSF pulled it's funding, University of Illinois Urbana-Champaign took up the cause of hosting (most) of the Geometry Center's web site (which was one of the first sites on the web when it started).
Starting points...
I, personally, think that with a little help these things can be understood... at least on a conceptual level. And a project was started back around 1990 to attempt to help people with visualizing this type of stuff. It started as an offshoot of the Minnesota Supercomputing Institute at the University of Minnesota called the Geometry Supercomputer Project. This was later given additional funding by the National Science foundation and the Department of Energy to form the Geometry Center.
My connection with the Geometry Center was rather limited... I did research there in the summer of 1994 on the topic of tight immersions of manifolds while I was a student at UCSD. I had the odd distinction of being the only person there that summer doing research that didn't make use of the computer systems at the center (about 8 SGIs, 12 Suns, 30 NeXTs, and 25 Macs) for my research.
But the Geometry Center put out some great stuff that was design to be approachable by everyone. A couple of these projects included video productions, which I think might be interesting for some of the people here. And thankfully even after NSF pulled it's funding, University of Illinois Urbana-Champaign took up the cause of hosting (most) of the Geometry Center's web site (which was one of the first sites on the web when it started).
Starting points...
Not Knot (1990)
This video is currently up on YouTube. You can find part one here and part two here. Additional information (including stills) can be found here.
Outside In (1994)
This video is also currently up on YouTube. You can find part one here and part two here. Additional information (including stills) can be found here. This video covers a topic close to the type of stuff I was researching in differential topology which included the study of immersions of manifolds and homotopy theory. As I recall, this video won some awards at SIGGRAPH and was made using a combination of Geomview, Mathematica and Renderman using an SGI Crimson RE (which was the highest end computer we had there back in 1994). Geomview was an application developed at the Geometry Center and originally only ran on SGI and NeXT systems (and I still run this app on my SGI and NeXT systems today).
Geometry and the Imagination (1991)
This was a course designed to introduce people (mainly teachers and math students) to some of the more interesting aspects of mathematics which you wouldn't normally see early on in a math education. You can find the original course materials here, and a more recent version here (pdf).
Hopefully this stuff will be both interesting and enjoyable for some of you guys.This video is currently up on YouTube. You can find part one here and part two here. Additional information (including stills) can be found here.
Outside In (1994)
This video is also currently up on YouTube. You can find part one here and part two here. Additional information (including stills) can be found here. This video covers a topic close to the type of stuff I was researching in differential topology which included the study of immersions of manifolds and homotopy theory. As I recall, this video won some awards at SIGGRAPH and was made using a combination of Geomview, Mathematica and Renderman using an SGI Crimson RE (which was the highest end computer we had there back in 1994). Geomview was an application developed at the Geometry Center and originally only ran on SGI and NeXT systems (and I still run this app on my SGI and NeXT systems today).
Geometry and the Imagination (1991)
This was a course designed to introduce people (mainly teachers and math students) to some of the more interesting aspects of mathematics which you wouldn't normally see early on in a math education. You can find the original course materials here, and a more recent version here (pdf).